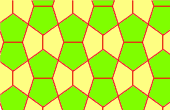
We paint different surfaces, such as a floor in a building or a pavement. It is obvious in tessellation, for example, it is expected that there would be no holes or overlapping tiles. In this way, the mathematical concept of tessellation is defined.

​

Definition: Tessellation refers to covering a surface with shapes, so that each surface is covered, with no voids and no overlapping of one shape on another. 

It is possible to sequence a plane only in geometric shapes and to achieve spectacular results. Such as the tessellation found in the Alhambra palace in Granada Spain, built in the 13th - 14th centuries by the Sultan of the Nasser dynasty.

More can be done. The Dutch artist, Maurits Cornelis Escher, was the first (as far as we know) to have developed a technique for paving the plane not only with geometric shapes but with paintings. Here are some examples:

In these paintings, it is possible to see how the line of one shape creates the line of the next, just as was done in the tessellation using geometrical shapes.

How could Escher draw these paintings? It seems that in order to paint them, he explored the plane's tessellation using geometrical shapes. Based on this exploration, he formulated rules for tessellating the plane, and thus succeeded in creating these works.